Indicators & Regimes
This chapter can also be downloaded as a pdf document
Spielauer, Martin, Thomas Horvath, Marian Fink, Gemma Abio, Guadalupe Souto, Ció Patxot and Tanja Istenič (2020) microWELT - Sustainability Indicators: The role of welfare state regimes National Transfer Accounts Network Working Paper 20-02 pdf
Abstract
This chapter discusses the indicators of the economic effects of population ageing developed in Chapter 4 from a comparative welfare state perspective. This analysis includes all four countries studied in the WELTRANSIM project (Austria, the UK, Finland, and Spain) representing the four different welfare state models in Europe. The results show that indicators based on disaggregated data can give a very distinct – and less pessimistic - picture of the economic effects of population ageing. While this is the case across all countries, the alleviating effects of composition changes driven by education expansion are particularly powerful in Spain. Based on aggregate data, Spain would be the country most hit by population ageing; when accounting for composition effects, it is moved to a comparatively favourable position. Substantial differences in indicators also arise in the UK. While less hit by population ageing and further educational improvements being modest, the high differences in income between education groups – and the liberal welfare state regime focusing on poverty prevention - amplify the alleviating effect of composition changes. Besides adding realism to projections of the impact of ageing on the economy, our approach also allows addressing and disentangling the role of different welfare state regimes and the effects of educational improvements.
Introduction
Dramatic changes in the Western world have characterised the past century. From an economic perspective, the extension of markets, globalisation, and technological progress have been the primary drivers of development. In parallel with these changes, there was a steady improvement in life expectancy coupled with a decline in birth rates, which has altered the population’s age structure. In this process, democratisation emerged, leading to the expansion of public education – a key element in the overall process – and other social policies, thus creating what we know nowadays as the welfare state.
These three axes of socio-political development (economic, demographic and political) are closely connected, although they evolved at different paces, and the interaction among them is not always clear. However, there seems to be a central element linked to each of these processes: the changes in family structures that have taken place over the last hundred years.
Along this process, the welfare state has been extending its action from mere monetary transfers for poverty reduction to more comprehensive programmes, such as providing basic social goods (education and health) and income substitution programmes, such as pensions, with a high insurance component. This process leads to the gradual substitution of private family intergenerational transfers which move to the public sphere and – to some extent - the market. Government intervention, in this case, goes beyond intra-generational redistribution, introducing intergenerational redistribution. A proper evaluation of the redistribution impact of welfare policies needs to consider the interaction of the three resource allocation devices along the lifecycle (family, public sector and markets). At the same time, understanding how these three resource allocation devices interact is crucial to face the current threats challenging the welfare state’s sustainability.
This research contributes to that goal by building and applying the dynamic micro-simulation model microWELT. microWELT produces results that allow for obtaining sustainability measures along the ageing process, disentangling the effects of the education transition, changes in family structures, and, at the same time, considering the impact of the welfare state design, starting from the education system. To this end, an analysis was carried out for four European countries, representative of the four welfare state regimes: Spain (Mediterranean), Austria (continental), Finland (Nordic) and the UK (Anglo-Saxon). This chapter’s primary focus is sustainability, with the following (chapters 7 and 8) focusing on analysing income redistribution issues.
The evaluation of sustainability will be based on the Support Ratio (SR) and the Impact Factor (IMP). SR started being a pure demographic measure, defined as the ratio between the working-age population (ages 16-64) and the total population. However, it has been extended in the literature to incorporate an economic dimension, measuring the relation between effective producers (or effective labour) and effective consumers. The term effective refers to the age shape of labour input and consumption used as weighting factors. NTA age profiles of labour income and consumption are especially suitable to compute these indicators.
Nevertheless, the SR ignores that even under these simplifying assumptions, the economy would be different, as changes in labour relative to capital input would alter wages and the interest rate. To overcome this shortcoming, Lee & Mason (2017) introduced some elements of general equilibrium into their projection capturing to some extent changes in factor prices (wages and interest rates) resulting from changes in the relative size of capital and labour. They also proposed another indicator (the Impact Index, IMP) which complements the SR and captures those changes in prices. The Impact Index measures the changing relation between resources available for consumption (total income, from labour and assets, net of savings), and effective consumers. This index can be computed both for a closed economy (changing wages and interest rates) and an open economy, giving further insights on the elements affecting sustainability.
This paper is organised as follows. Section 2 gives an overview of the microWELT model and its application from the welfare state regimes’ perspective. It also introduces the NTA approach and the disaggregated NTA data used for our analysis. This part is kept very short as extensive documentation of model and data are available in separate papers (Spielauer et al. 2020a/b, Abio et al. 2020). Section 3 presents the calculation and projection of the sustainability indicators computed (the Support Ratio and the Impact Index) and the related analysis for the four countries studied. The final Section is left for final remarks. Definitions of all the variables of the model are collected in an Appendix.
The microWELT model and the welfare regimes
The most well-known typology of welfares states (initiated by Esping-Andersen, 1990) identifies three welfare regimes: conservative (Continental European), liberal (Anglo-Saxon), and social-democratic (Nordic), based on the concepts of de-commodification and social stratification. The latter refers to its redistributive potential (mainly at the intragenerational level). De-commodification is a more complex concept: the extent of the immunisation from market dependency, which turns out to be deeply connected with the NTA approach. Quite intuitively, the extent of independence from the market relies on the availability of alternative resource allocation devices, namely the public sector and the family. As discussed in Chapter 8, the degree of familiarisation of the welfare state was incorporated as a key concept in the posterior literature, and NTA estimations allow to measure it.
The initial classification of Esping-Andersen (1990) was extended by Leibfreid (1993) and Ferrera (1996), who claimed for the need to differentiate Mediterranean countries (Spain, Portugal and Italy) from the Continental regime. Following this extension led to selecting four countries representative of the four welfare state regimes, to perform our analysis. Many authors contributed to consider additional welfare domains in the Esping-Andersen initial analysis. One key feature of welfare models not highlighted in the Esping-Andersen’s work is the quality of the education system. Education is crucial for the later individuals’ outcomes (West & Nikolai, 2013) and, therefore, an important feature to be analysed when discussing differences in the welfare regimes. microWELT incorporates education as the key socioeconomic variable, which affects the economic emancipation of young adults, partnership formation, fertility, family type, and eventually, mortality.
In microWELT agents are heterogeneous in terms of educational attainment. The model parametrisation starts from the observed distribution in the base year and from estimated patterns of intergenerational transmission of education (see Figure 7 and Table 1 in Chapter 2, respectively). The estimation results indicate that the impact of parents’ education on the transition from low to medium education is the weakest in Finland and the highest in Spain, Austria and the UK falling in-between. The ranking of countries changes a bit when analysing the transition from medium to high education. However, Finland maintains its position, followed by Austria, Spain, and the UK. Accordingly, in Finland, the young have the most universal education opportunities, a central characteristic of Nordic welfare states. Nordic countries are characterised by high public expenditures on education, resulting in high reading scores at the age of 15, and a lower proportion of early leavers from education (West & Nikolai, 2013). Our estimations also reveal the characteristics of the liberal welfare countries, where public expenditures for education are high especially at primary levels, whereas not so much at later educational levels (West & Nikolai, 2013). When analysing the transition from medium to high education, the universal rights in the UK disappear. Still, the transition rates from medium to high education are lower in Austria and Spain as compared to the UK. This can be explained by the socially stratified schooling system, with high academic selection in Continental and Mediterranean countries. While in Mediterranean countries, the selection happens only later in school life, it comes earlier in Continental countries (West & Nikolai, 2013). The high academic selection already at younger ages in Austria explains the much lower share of highly educated in Austria as compared to the other analysed countries.
Our projections based on the current intergenerational transmission (Figure 8 in Chapter 2) result in very different changes in the educational composition among the four countries. Finland and the UK show the smallest changes, while Austria and especially Spain show the most significant changes. There is still a substantial increase in the share of the highly educated at the expense of the medium for the former. For the latter, there is still a substantial reduction in the lower educated.
microWELT’s parametrisation incorporates childlessness by education level where there are sizable differences for all countries (see Figure 3 in Chapter 2). UK and Finland have the lowest level of childless women, while Finland has experienced a reversal in the education gradient. For cohorts born after the 1950s in Finland, the low educated childlessness has been increasing, overcoming the other two education levels. While investigating the causes for these trends and their interaction with partnership formation is beyond the scope of this paper, it is crucial to understand the interaction between the design of the welfare state, including education outcomes, and the evolution of fertility, which in turn affects the sustainability of the welfare state itself.
Interestingly, Esping-Andersen and Figari (2015) find some signs of a reversal in the education gradient of fertility, which could have been led by reaching a more gender-egalitarian partnership mode, accompanied by policies. The model presented here takes the fertility observed in 2010 as a starting point and reproduces age-specific fertility rates reproducing EUROSTAT projections. The initial values highlight the paradox that fertility stayed relatively high in countries with very different social protection levels like the UK and Finland (with fertility rate at 1.9 in 2010). Nevertheless, Finland has experienced a dramatic drop in fertility rates since 2010, which was not observed in the UK. On the other hand, the Mediterranean and conservative countries seem to be trapped in low fertility levels (1.3 in Spain and 1.4 in Austria respectively in 2010). This even though the family’s traditional role is emphasised in Mediterranean countries like Spain; and the state extensively supports motherhood, especially in conservative countries like Austria.
Another modelled process affected by education is the age of economic emancipation and leaving home, which also has a potential link with the welfare regimes’ typology. While children in social-democratic countries, like Finland, leave their home earlier (at age 21.8 on average), Spain is the country in which young people leave home the latest (in the late 30s, relying more on the family (see Figure 12 in Chapter 2). The UK and Austria stay in between, with children in the UK leaving their home earlier (24.6 compared to 25.4 in Austria). This is in line with the liberal welfare characteristics, where non-dependent individuals rely to a greater extent on the market than in other countries.
Education (besides age, the presence of children in the family, and the youngest child’s age) also impacts the likelihood of a woman to live in a partnership. In the simulation, partners are matched by observed distributions by age and education. Persons are linked to nuclear families. These links are maintained responding to events such as union formation, new partnerships, leaving home, and death. We found pronounced differences in partnership patterns, some features linkable to welfare state regimes. Examples are the very high proportion of lone mothers among young mothers with low education in the UK; in contrast, single parents are frequently observed in Finland at higher ages of children.
Finally, we model both projected mortality improvements reproducing EUROSTAT projections, while simultaneously accounting for the mortality differentials by education. All studied countries have sizable differences in mortality by education level, which impact both the sustainability and the redistributive effects of public pension systems.
The model implements a parallel parameterisation of NTA variables by three levels of disaggregation: by age, by age and sex, and by age, sex, education and family type. Also, microWELT implements individual longitudinal accounts and mechanisms to balance national accounts over time. Figure 1 shows the NTA age profiles introduced in microWELT to analyse sustainability: labour income and total consumption age profiles by education level (for children and students up to 25, education refers to parents’ education). Labour income profiles show the typical inverted U-shape, with income concentrated on working ages. The high-educated reach higher incomes, especially later in their work career, and work until older ages. At the peak, the high education group’s average labour income is much higher than for the low educated. The average income for ages 30 to 49 is 3.42 times higher at the high education level for the UK, while it is 2.73, 2.16 and 2.11 in Austria, Spain and Finland, respectively.
Consumption age profiles are much smoother than labour income. Moreover, the differences between education groups are smaller, especially in Finland, due to a stronger redistributive policy. The UK consumption profiles seem to converge at the end of life, but this is because the education level cannot be appropriately identified in the consumption survey. Consumption profiles are flatter when using aggregate NTA data, whereas the consumption profiles disaggregated by education indicate different patterns. This is a consequence of the different educational compositions in the various age groups and illustrates one of the problems in applying the current aggregated cross-sectional profiles in the future.
Figure 1: NTA age profiles of labour income and consumption
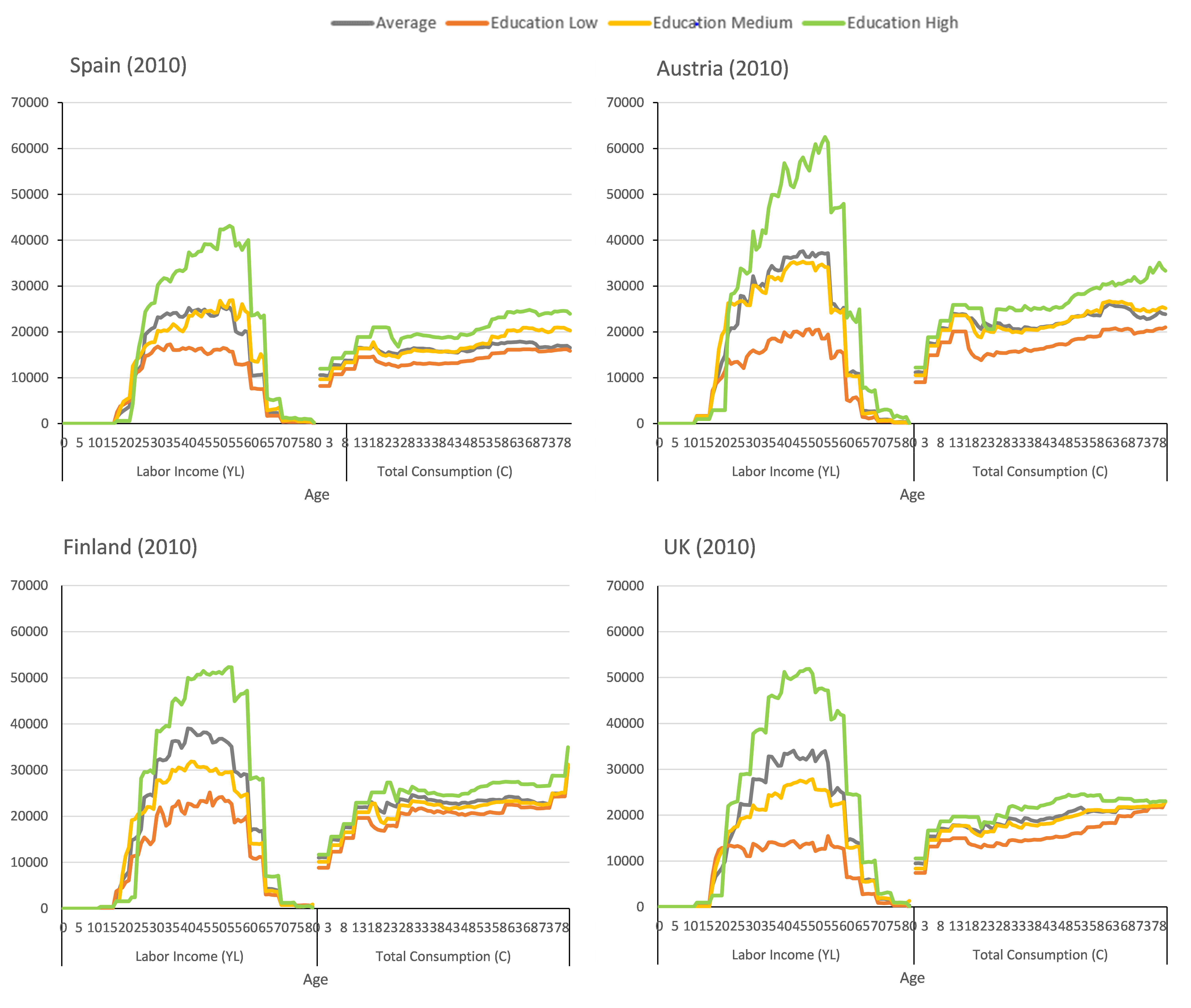
Source: Simulation output based on NTA data by education and family type developed in the WELTRANSIM project.
Measuring sustainability
Population ageing affects the proportion between the active and the economically dependent population. Besides decreasing fertility and mortality, in several countries, the post-war baby boom and the subsequent baby bust created a kind of demographic cycle that enlarged the otherwise gradual ageing process. The effect of baby-boomers reaching retirement age is further aggravated by declining mortality. Nevertheless, education improvements and the increasing labour force integration of women, as well as health improvements and policy reforms leading to an increase in retirement age, can potentially mitigate this effect. In other words, demographic measures, such as the purely age-based dependency ratio (the population considered being in dependent age divided by the population in working age where working age is set to, e.g. 18-64) might not adequately capture the burden of population ageing on the economy. Specifically, the dependency ratio is insensitive to changes in labour market outcomes and policies. This effect is addressed by indicators based on National Transfer Accounts (NTA).
The most widely used indicator based on the NTA approach is the economic Support Ratio (SR) measuring the changing relation between effective producers (or effective labour) to effective consumers. It builds on the demographic support ratio, which in its simplest form relates the working-age population (e.g. 16-64) to the total population. Applying weights to more accurately represent producers and consumers – e.g. by accounting for labour force participation – was first suggested by Cutler et al. (1990; for further discussions on the definition of support ratios see Mason and Lee (2006), Patxot et al. (2011)).
The economic Support Ratio (in the following SR) gives a more comprehensive measure by replacing the size of the working-age population by the population, weighted by the age profile of the current average labour income. Similarly, the total population size is replaced by the population, weighted by the age profile of current consumption. Projections of the SR are available for many countries. When the Support Ratio increases, economies experience the so-called “demographic dividend” (a relative increase of the working-age population with respect to the dependent population).
As said above, education expansion is one of the sources that might mitigate the impact of ageing. Rentería et al. (2016) propose a decomposition of the demographic dividend into age and education effects using NTA age profiles by educational attainment. Their results confirm that the education component partly offsets the future negative effect of ageing on the Support Ratio in Spain. The computations here extend this line of research, including not only the changing education composition but also changes in family structures. While still being a cross-section measure ignoring all trends within the distinguished population groups, incorporating the education and family dimension adds some realism by accounting for critical composition effects. On average, higher educated people obtain higher income, stay in the labour force longer, and – at active age – pay higher taxes and contributions. On the other side, they also live longer and receive higher pensions.
SR aims at providing an indicator of how the ratio of labour income and consumption (both age-weighted) would be different in a world which besides the age distribution (and in our case education and family type) looked exactly like the world today. However, it ignores that even under these simplifying assumptions, the economy would be different, as changes in labour relative to capital input would alter wages and the interest rate. To overcome this shortcoming, Lee & Mason (2017) introduced some elements of general equilibrium into their projection, capturing to some extent changes in factor prices (wages and interest rates) resulting from changes in the relative size of capital and labour. They also proposed another indicator (the Impact Index, IMP) which complements the SR and captures those changes in prices. The Impact Index measures the changing relation between resources available for consumption (total income, from labour and assets, net of savings), and effective consumers. The calculation the IMP is affected by wages and interest rates resulting from a simple aggregate production function. Hence, this index can be computed both for a closed economy (changing wages and interest rates) and an open economy (constant wages and interest rate).
The Support Ratio (SR)
The Support Ratio shows the relationship between available labour income (effective labour) to the current consumption level (effective consumers). The microWELT model is used to project the evolution of the SR, keeping constant the per age profiles of labour income and consumption observed in the base year (2010).
Figure 2 shows the results obtained for the evolution of the two components of the SR: effective labour (L) and effective consumption (C), in the four countries analysed. Effective labour is simply the age profile of labour income for the base year, as depicted in Figure 1, re-weighted by projected population numbers, set into relation to the initial 2010 value. Effective consumers (N) are calculated based on the age profiles of total consumption in Figure 2. All magnitudes are expressed as an index set to 1 in the base year.
Results based on the disaggregated NTA data developed in the WELTRASNIM Project (by education and family structure) are compared with those obtained using traditional NTA data, only by age. As observed, composition changes affect both the effective labour and effective consumption. The evolution of L and N using aggregated NTA age profiles shows changes in demography, which are quite substantial. As seen in Figure 1 in Chapter 2, the demographic projections employed imply a long period of births below deaths in all countries except the UK. This gap is closed by migration flows in Austria and Spain, so that the total population stays almost constant. In Finland, the gap is not closed, and the population declines slowly. In the UK, the total population increases.
Effective workers decrease in all countries due to the ageing process, except in the UK, where fertility stays high, and births are always higher than deaths. The decrease in L is stronger in Spain and Finland. Using disaggregated NTA, i.e., considering the positive effect of the education transition completion and other composition effects, mitigates the decrease in effective producers so that there is an increase for some years in Spain and Austria. In the UK, accounting for composition effects produces a higher increase in effective producers. Concerning effective consumers, the ageing process leads to an increase in all countries, moderated by composition effects when disaggregated NTAs are used. The difference is smaller than in effective producers, as are the differences in the consumption profiles. Overall, the effects of (not) ignoring composition effects are considerable: e.g. for Spain, changes in effective consumers are more than double as high when basing the calculation on disaggregated NTAs.
Figure 2: Effective Labour (L) and Effective Consumers (N)
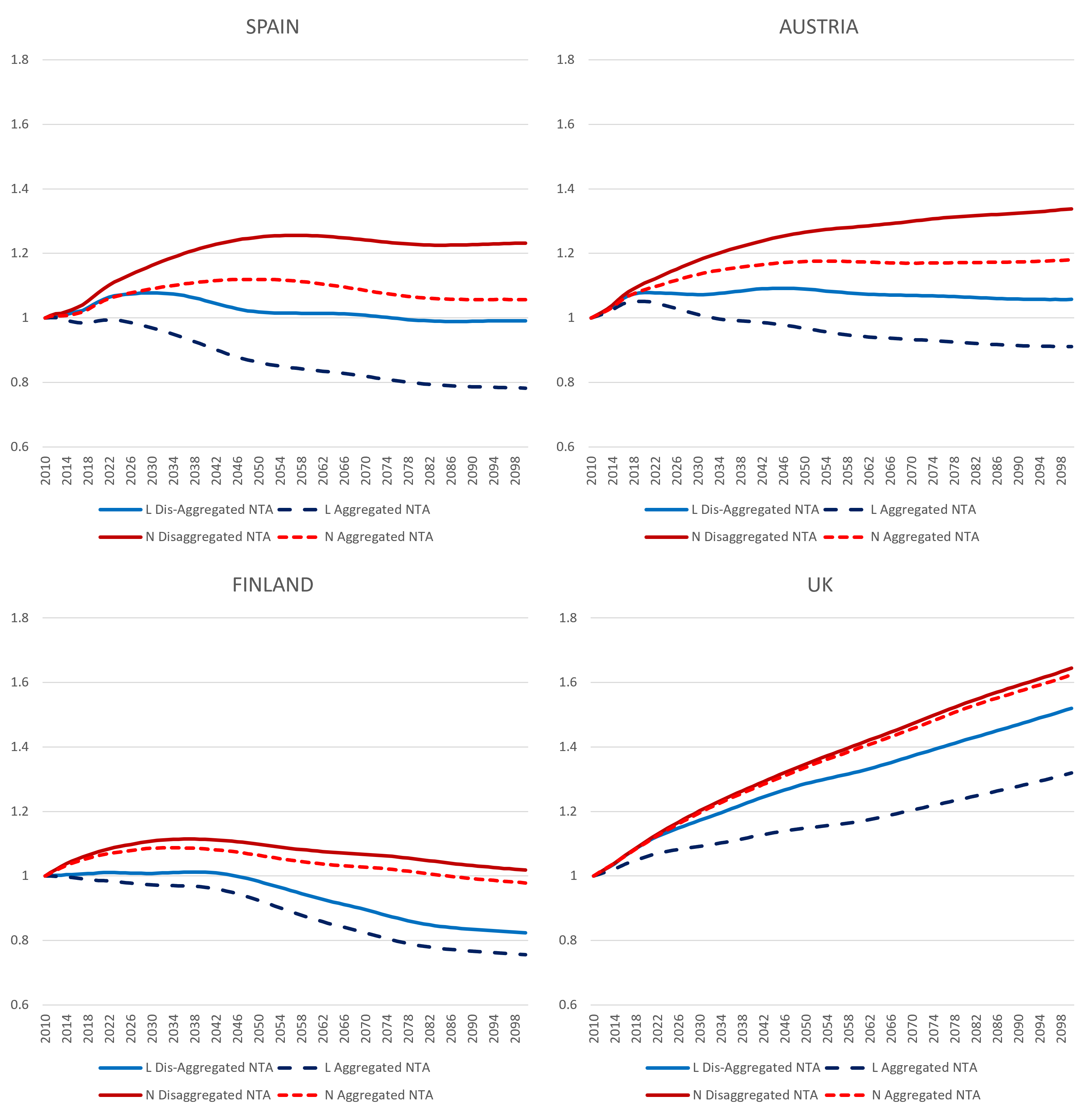
Source: Simulation output based on NTA data by education and family type (WELTRANSIM project) compared to outcomes based on aggregate NTAs (AGENTA project).
Figure 3 shows the evolution of the Support Ratio, obtained by dividing effective producers (L) by effective consumers (C). Again, the results obtained with disaggregated NTA (by age, level of education and family type) are presented against those resulting from standard NTA profiles (only by age). Additionally, two more measures are provided. First, a pure demographic index built as the ratio of population 18-64 to the total population. Second, an index relating the changes in effective labour based on disaggregated NTA to the change in effective consumers based on aggregated NTA. This last indicator measures to which extent today’s consumption by age can be maintained accounting for the changing education composition of the population, without maintaining the consumption standards within each education group. Results show that the changing population composition by education improves the situation but does not outweigh the ageing effect. For the UK, this indicator is almost the same as the one based in disaggregated NTAs due to the difficulties mentioned above in identifying consumption by education level.
Figure 3: Support Ratios
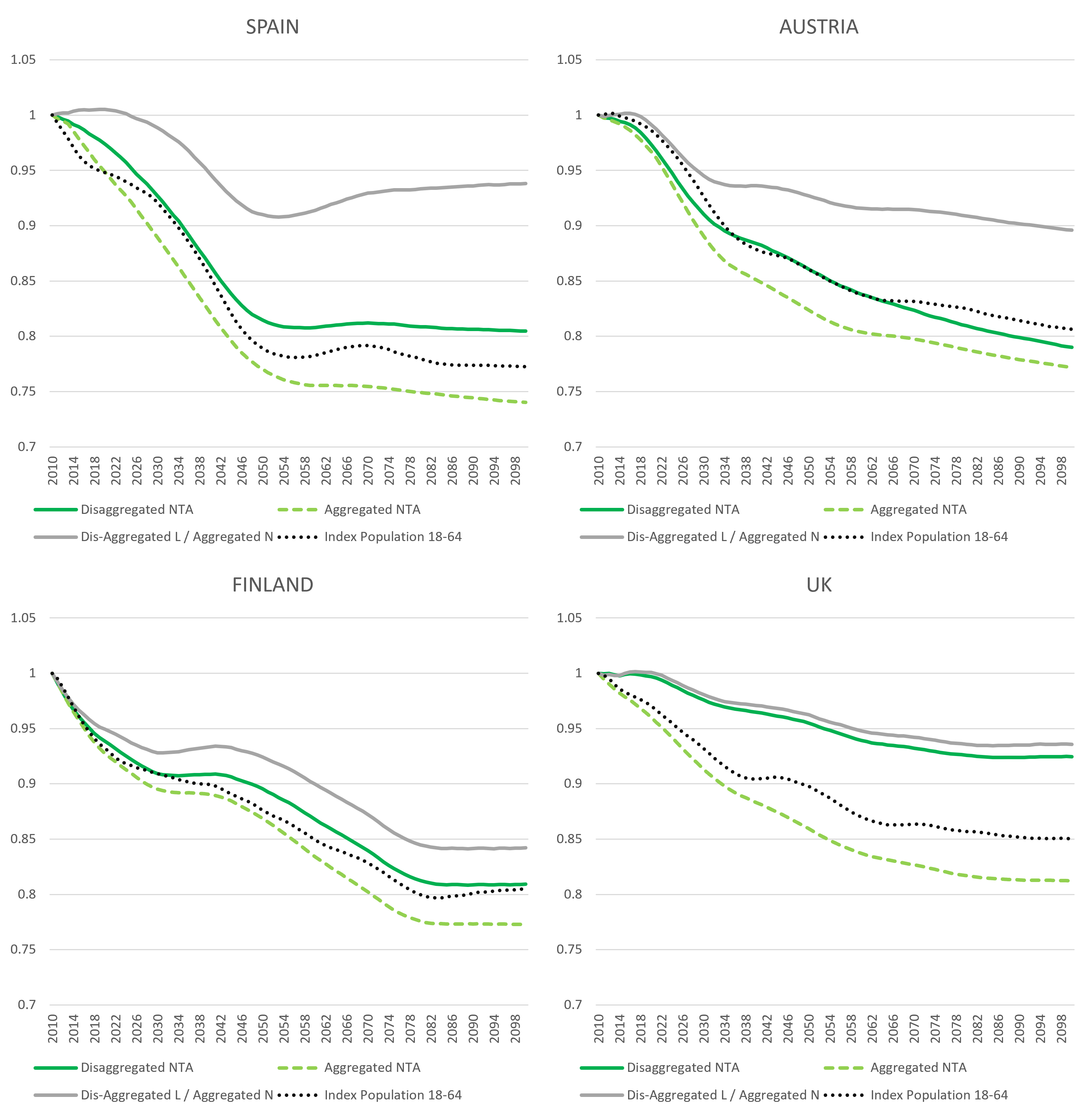
Source: Simulation output based on NTA data by education and family type (WELTRANSIM project) compared to outcomes based on aggregate NTAs (AGENTA project).
Comparing the four indicators in Figure 3 reveals some of the problems of an indicator based on aggregate NTAs.
NTA literature promotes the Support Ratio as a more realistic indicator of the effect of population ageing compared to purely demographic measures based on the relation of age groups. They find that for Europe, the Support Ratio gives a more dramatic outlook than purely demographic measures (Prskawetz & Sambt 2014). This present study shows that, when accounting for composition effects, these results can be reversed. Also, the analysis herein indicates similar trends, while the size of these results is very country-specific.
NTA measures based on aggregate NTAs not only ignore improvements in human capital (also pointed out by Rentería et al., 2016). The indicator is also based on average age-specific consumption. As an indicator of the extent to which the living standard of a population can be maintained, it refers to the average population by age and not to the maintenance of the relative consumption standards as observed today for given population groups by education and other characteristics. The results show that when using aggregate consumption by age as the standard reference, a much higher level of consumption can be maintained due to composition effects: for Spain, in the long run, the SR reaches 94% of its initial value compared to 75% indicated by the Support Ratio based on aggregated NTAs.
The disaggregated NTA developed in the WELTRANSIM Project do not only distinguish by education, but also by family type. The rationale is to provide measures which allow for the distributional study not only by education but also by family structure, especially between people with and without children. As a tool for comparative studies, including a longitudinal perspective, microWELT projections account for longevity differentials by education. In order to assess to which extent such detail not only allows distributional studies but also impacts summary measures such as the Support Ratio, two what-if scenarios were created:
In Scenario A, “switched off” is the relative mortality differences by education. This scenario does not affect overall age-specific mortality, but who – at a given age and for given sex – dies. This scenario thus ignores that people with higher education on average not only consume more but also live longer lives.
In Scenario B, the effect of the differences in the distribution of family sizes by education (the concentration of reproduction; see Spielauer, 2005 and Shkolnikov et al., 2004) on the Support Ratio is studied. While microWELT accounts for differences in timing and quantum of fertility by education, in this scenario the assumption is a low concentration of reproduction setting childlessness to a very low level (of 5%) regardless of education and applying the same fertility patterns to all education groups. This scenario does not affect overall age-specific fertility rates but distributes children more evenly among women.
Figure 4 depicts the effect of mortality and fertility differentials by education on the Support Ratio. In both scenarios, the effects are limited, since total mortality and fertility are kept in line with the baseline population projections, but still noteworthy. As expected, ignoring longevity differentials would lead to a smaller decline in the Support Ratio in the four countries. This reflects the more significant contribution of highly educated individuals in terms of higher wages and larger contributions to the public coffers (see Chapter 7). The effect of the concentration of reproduction is of comparable magnitude or lower, but in the case of Austria and the UK it goes in the opposite direction. The size of the effect is larger in countries where the differences in childlessness by education level are bigger (Austria and Spain – see Figure 3 in Chapter 2).
Figure 4: The effect of mortality and fertility differentials on the Support Ratio

Source: Simulation output based on NTA data by education and family type developed in the WELTRANSIM project.
The Impact Index (IMP)
In contrast to the Support Ratio, the Impact Index is a broader measure which contains more information and permits considering, to some extent, changes in wages and interest rates resulting from the changing relation between labour supply and capital due to demographic change. The Impact Index measures the change in the relationship between resources available for consumption and the consumption level needed to keep the initial standards. As described above (see also Appendix I), compared to the Support Ratio, the Impact Index replaces “effective producers” (a measure for labour input) in the numerator with the consumption which would be possible given the available income. This way, new information measuring the gap between income available for consumption -assuming a constant age-specific saving rate- and initial consumption standards is thus added. We also gain information by accounting for changes in wages and capital income (in the closed economy case).
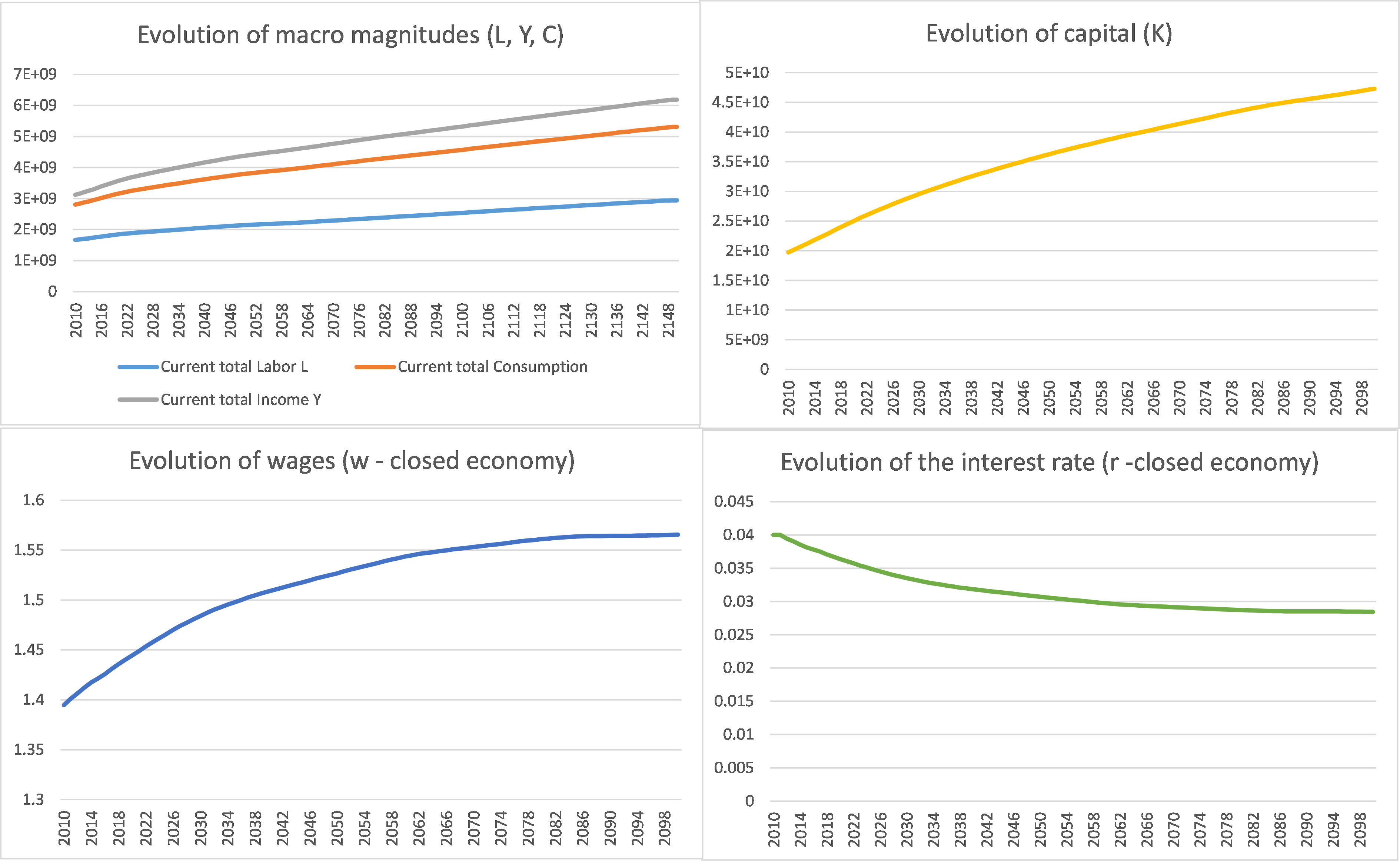
As in ageing societies, labour force becomes relatively scarcer. In a closed economy, interest rates decrease while wages increase, the two price changes affecting the consequences of ageing on future consumption in opposite directions. While the negative effect of lower interest rates on consumption - compared to the alleviating effects of higher wages - is almost negligible for Austria and Finland, it is strong for the UK and Spain (see Appendix II for the evolution of the macro magnitudes). Calculation of future production as a function of capital and labour input requires the choice of a production function. The Impact Index applies a Cobb Douglas, assuming for simplicity that the productivity growth is null. Besides the information required to calculate the Support Ratio, the only additional information required is an initial interest rate (as in Lee & Mason 2017, used here is 4% for the illustrations). NTAs do not contain information on capital stocks. As a proxy, initial capital endowment by age is calculated based on the assumed interest rate and kept constant in the simulation. In contrast, in the closed economy case wages and interest rates adapt to population change. While the saving rate is used for calculating consumption, there is no longitudinal consistency between savings and the stocks of capital, which are entirely based on today’s estimated stocks by age. (See Sánchez-Romero et al. (2013) for an alternative approach which fully captures the general equilibrium macroeconomic effects at the cost of less disaggregation.) The equations behind the model calculations of the Impact Index are presented in Appendix I.
Figure 5 shows the Impact Index comparing simulations based on aggregated versus disaggregated NTA data and contrasting these indicators to Support Ratios previously obtained. The effects are considerable, both (1) accounting for the gap between consumption needs and total available income net of savings, and changes in prices compared to the SR, and (2) basing the analysis on disaggregated data. For Spain, assuming an open economy, in the long run, 93% of consumption could be maintained when using disaggregated data, compared to 80% when using aggregate data. In the UK, the effects are of similar magnitude, while for Austria and Finland, they are similar in direction but smaller.
Figure 5: The Impact Index compared to the Support Ratio
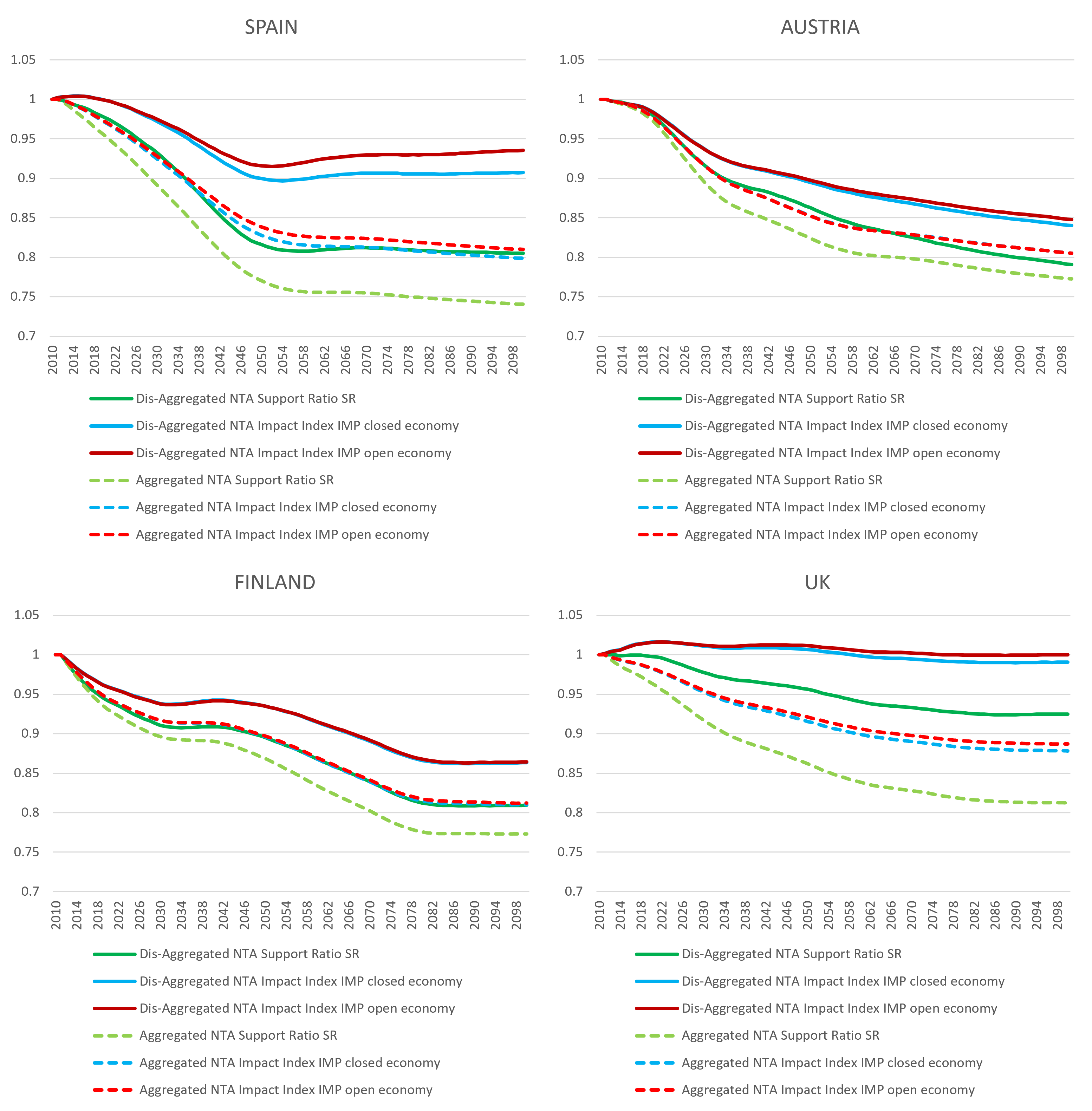
Source: Simulation output based on NTA data by education and family type (WELTRANSIM project) compared to outcomes based on aggregate NTAs (AGENTA project).
Basing the Impact Index on disaggregated NTA alleviates the burden of population ageing through different channels. On the one hand, a different kind of composition effects arise: the simple mechanics behind the calculation of the Impact Index leads to a much higher increase in available capital. This is due to the fact that capital endowment by age and education is assumed to be constant, and observed endowments are considerably higher for higher educated people, driving up capital stocks by composition effects. On the other hand, price effects act so that in the open economy case, income available for consumption is higher compared to a closed economy. The latter effect is particularly strong in Spain and the UK, leading to a situation where a closed economy (with lower interest rates and higher wages) would result in a higher decrease of the Impact Factor than in an open economy.
Both the Support Ratio and the Impact Index take effective consumers (N) in the denominator. As the number of effective consumers changes when applying disaggregated NTA, this thus also affects the Impact Index and its interpretation: using aggregated NTA, how future consumption is related to today’s consumption by age only is expressed. Using disaggregated NTA, future consumption is related to consumption accounting for the differences in consumption levels between education groups. In Figure 6, three versions of the closed economy Impact Index are compared, calculated by different combinations of aggregated and disaggregated NTAs. Relating the future consumption based on disaggregated NTA to today’s consumption based on aggregated NTA, in the Spanish case (and in the UK to a lower extent), the most favourable version of the Impact Index stays above or close to 1 over the whole projection horizon. Changes in the education composition thus, compensate for the effect of ageing when considering changes in consumption only by age. For Austria and Finland, this variant of the Impact Index also declines over time, whereas far less than other variants.
Figure 6: The Impact Index compared to the Support Ratio for different variants of aggregation
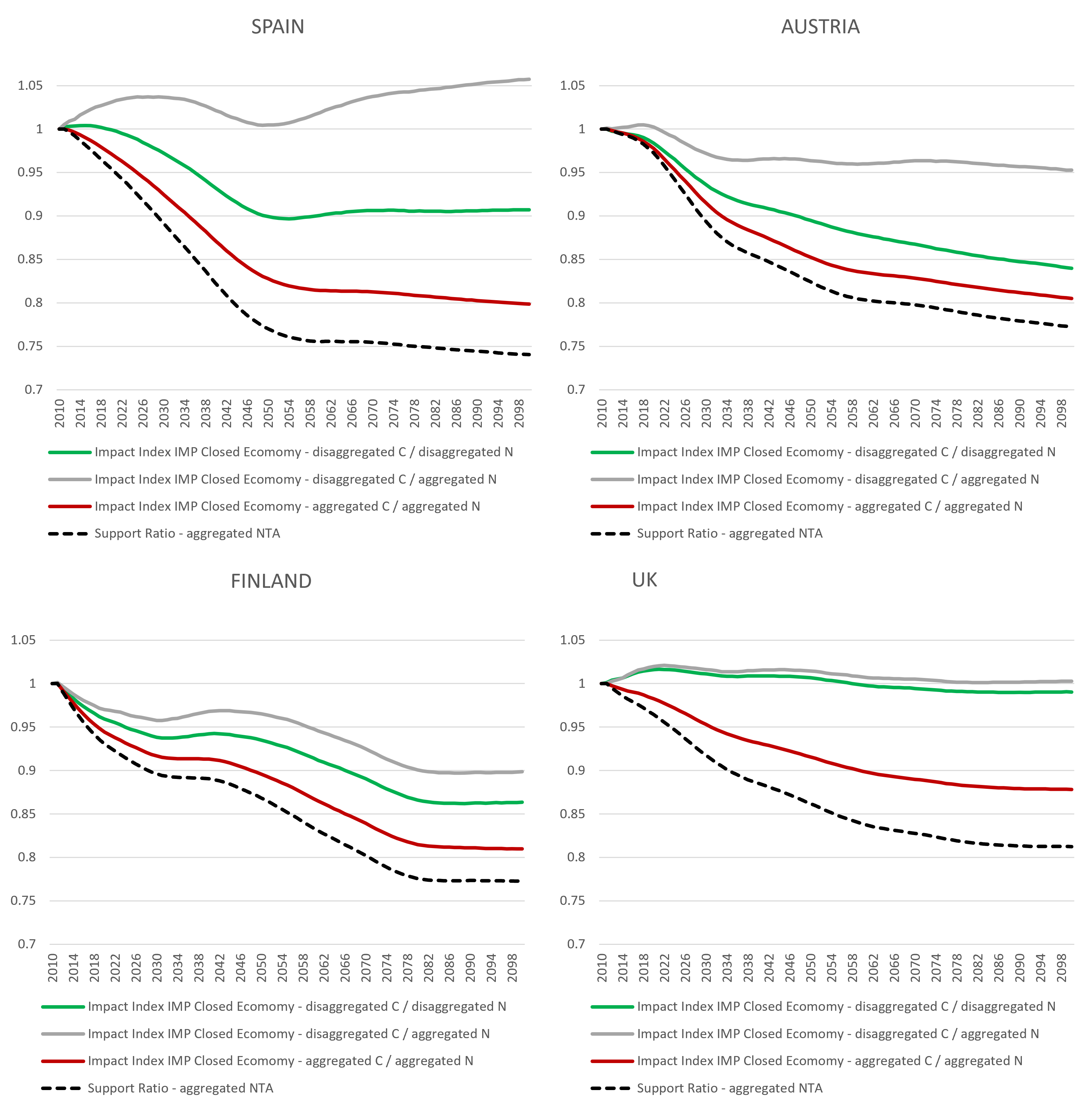
Source: Simulation output based on NTA data by education and family (WELTRANSIM project) compared to outcomes based on aggregate NTAs (AGENTA project).
Discussion
Population ageing is one of the main challenges that current societies are facing. In particular, the sustainability of the welfare state transfers, consolidated in most advanced countries along the second half of the 20th century, is nowadays questioned, as they are highly dependent on the population age structure.
This paper has studied how changes in the population composition, not only by age but also by education and family characteristics, impact welfare state sustainability. To that end, two indicators of the economic effects of population ageing based on National Transfer Accounts (NTA) have been projected and analysed. On the one hand, the economic Support Ratio (SR), measures the ratio between effective labour and effective consumers, weighting the number of workers and consumers by the corresponding NTA age profiles of labour income and consumption. On the other hand, a second indicator named Impact Index (IMP) tries to consider possible changes in wages and interest rates resulting from the variation of the demographic structure.
The Support Ratio was introduced to overcome the shortcomings of a pure demographic measure of economic dependency, based entirely on age, claiming that applying age profiles of economic variables (based in NTA) as observed today, can give a more realistic outlook of the economic burden of ageing. In practice, for most European countries, the economic SR showed more severe effects of ageing than the pure demographic indicator. The Impact Index adds a simple economic model to the calculation of an indicator of the burden of ageing, introducing wage and interest rate reactions.
However, our results show that accounting for the composition effects introduced by education expansion (by using disaggregated NTA data), changes these results considerably. Except for Austria, the SR calculated with disaggregated NTA stays above the purely demographic measure. The difference is especially high in Spain, which has the strongest demographic ageing process – but also considerable improvements in education. Substantial differences in indicators also arise in the UK. While less hit by population ageing and further educational improvements being modest, the high differences in income between education groups – and the liberal welfare state regime focusing on poverty prevention - amplify the alleviating effect of composition changes. Besides adding realism to projections of the impact of ageing on the economy, our approach also allows addressing and disentangling the role of different welfare state regimes and the effects of educational improvements.
References
Abio, Gemma, Patxot, Concepció, Souto, Guadalupe, Istenič, Tanja (2020), Disaggregated National Transfer Accounts by Education and Family Types for Spain, UK, Austria, and Finland. University of Barcelona, forthcoming. The paper will be made available at the project website www.weltransim.eu
Cutler, D.M., Poterba, J.M., Sheiner, L.M., Summers, L.H. (1990), An Aging Society: Opportunity or Challenge? Brooking Papers on Economic Activity 1990(1): 1–73.
Doepke, M., Tertilt, M. (2016), Families in Macroeconomics, Handbook of Macroeconomics, in: J. B. Taylor & Harald Uhlig (ed.), Handbook of Macroeconomics, edition 1, volume 2, chapter 0, pages 1789-1891, Elsevier.
Esping-Andersen, G., Figari, FC (2015), Re-theorizing Family Demographics, Population and Development Review 41(1): 1-31.
Esping-Andersen, G. (1990). The three worlds of welfare capitalism. Princeton: Princeton University Press.
Lee, Ronald, Mason, Andrew (2017), Some Economic Impacts of Changing Population Age Distributions - Capital, Labor and Transfers. Paper prepared for the 2017 World Congress of the IUSSP in Cape Town. https://iussp.confex.com/iussp/ipc2017/mediafile/Presentation/Paper2638/MacroImpactCapetown_v3.pdf.
Lee, Ronald, McCarthy, David, Sefton, James, Sambt, Jože (2017), Full Generational Accounts: What Do We Give to the Next Generation? Population and Development Review 43(4): 695–720.
Mason, A., Lee, R. (2006), Reform and support systems for the elderly in developing countries: Capturing the second demographic dividend, GENUS 52(2): 11–35.
Patxot, Ció, Renteria, Elisenda, Sánchez-Romero, Miguel, Souto, Guadalupe (2011), Integrated results for GA and NTA for Spain: some implications for the sustainability of welfare state, Moneda y Crédito, 23:7-51.
Prskawetz, Alexia, Sambt, Jože (2014), Economic support ratios and the demographic dividend in Europe - Demographic Research: Volume 30, Article 34.
Rentería, Elisenda, Souto. Guadalupe, Mejía-Guevara, Iván, Patxot, Ció (2016), The effect of education on the demographic dividend, Population and Development Review, 42, 4, 651-671.
Sánchez-Romero, M., Patxot, C., Rentería, E., Souto, G. (2013), On the Effects of Public and Private Transfers on Capital Accumulation: Some Lessons from the NTA Aggregates, Journal of Population Economics, 26: 1409-1430.
Shkolnikov, Vladimir, Andreev, E.M., Houle, Rene, Vaupel, J.W. (2004), The concentration of reproduction in cohorts of US and European women. Max Planck Institute for Demographic Research WP-2004-027. http://www.demogr.mpg.de/papers/working/wp-2004-027.pdf.
Spielauer, Martin, Horvath, Thomas, Fink, Marian (2020a), microWELT: A Dynamic Microsimulation Model for the Study of Welfare Transfer Flows in Ageing Societies from a Comparative Welfare State Perspective. WIFO Working Paper 2020.
Spielauer, Martin, Hyll, Walter, Horvath, Thomas (2020b), microWELT: Socio-Demographic Parameters and Projections for Austria, Spain, Finland and the UK WIFO Working Paper 2020.
Spielauer, Martin (2005), concentration of reproduction in Austria: general trends and differentials by educational attainment and urban-rural setting. In: Vienna yearbook of population research 2005.
Sutherland, Holly, Figari, Francesco (2013), EUROMOD: The European Union tax-benefit microsimulation model. International Journal of Microsimulation. 6. 4-26. 10.34196/ijm.00075. https://microsimulation.org/IJM/V6_1/2_IJM_6_1_Sutherland_Figari.pdf.
United Nations (2013), National Transfer Accounts Manual: Measuring and Analysing the Generational Economy. United Nations Publications. https://www.un.org/en/development/desa/population/publications/pdf/development/Final_March2014.pdf.
West, A., & Nikolai, R. (2013). Welfare regimes and education regimes: Equality of opportunity and expenditure in the EU (and US). Journal of Social Policy, 42(03), 469-493.
Appendix I: Calculation of the Support Ratio and the Impact Index
Variables
yl(x) Average labour income age x
yk(x) Average capital income age x
P(x) Population age x
i(x) Average savings age x
s(x) Saving rate age x - constant
c(x) Average consumption age x (reference values for calculation of N)
L Labour
K Capital
I Saving
S Saving rate
C Consumption
r Interest rate
w Wage rate
Yl Labour Income
Yk Capital Income
Y Total Income Yl+Yk
α Alpha – constant
N Effective Consumers (population-weighted base-year consumption)
l(x) Average labour age x – constant
k(x) Average capital age x – constant
Cobb Douglas Production Function
Y = L^α K^(1- α)
Y = wL + rK
w = αY / L
r = (1- α) Y / K
Yl = wL = αY
Yk = rK = (1- α)Y
Known
yl(x) NTA data of reference year
yk(x) NTA data of reference year
i(x) NTA data of reference year
c(x) NTA data of reference year
P(x) NTA data of reference year
r parameter for reference year (used also to estimate the capital stock)
Calculated for initial year
Yl = ∑ yl(x) * P(x)
Yk = ∑ yk(x) * P(x)
Y = Yl + Yk
α = Yl / Y
K = Yk / r
L = ( Y / K^(1- α))^(1/α)
w = Yl / L
s(x) = i(x) / (yl(x) + ykx))
l(x) = yl (x) / w
k(x) = yk (x) / r
N = ∑ cl(x) * P(x)
Simulation: calculate for an updated population by age P(x)
(a) Closed Economy
L = ∑ l(x)* P(x)
K = ∑ k(x)* P(x)
Y = L^α K^(1- α)
Yl = αY
YK = (1- α)Y
w = αY / L
r = (1- α) Y / K
N = ∑ c(x) * P(x)
yl(x) = w * l(x)
yk(x) = r * k(x)
C = ∑ (1-s(x)) * (yl(x) + yk(x) ) * P(x)
(b) Open Economy (difference to closed)
r is given exogenously (and assumed constant in the simulation)
w = (Y – r * K) / L (following directly from the identity Y = w * L + r * K)
Yl = w * L
YK = r * K
Indices
SR = L / N Support Ratio (same for open and closed economy)
IMP = C / N Impact Index (different for closed and open economy)
Appendix II: Evolution of the macro magnitudes, wages and interest rates
a) Spain (base scenario, closed economy)
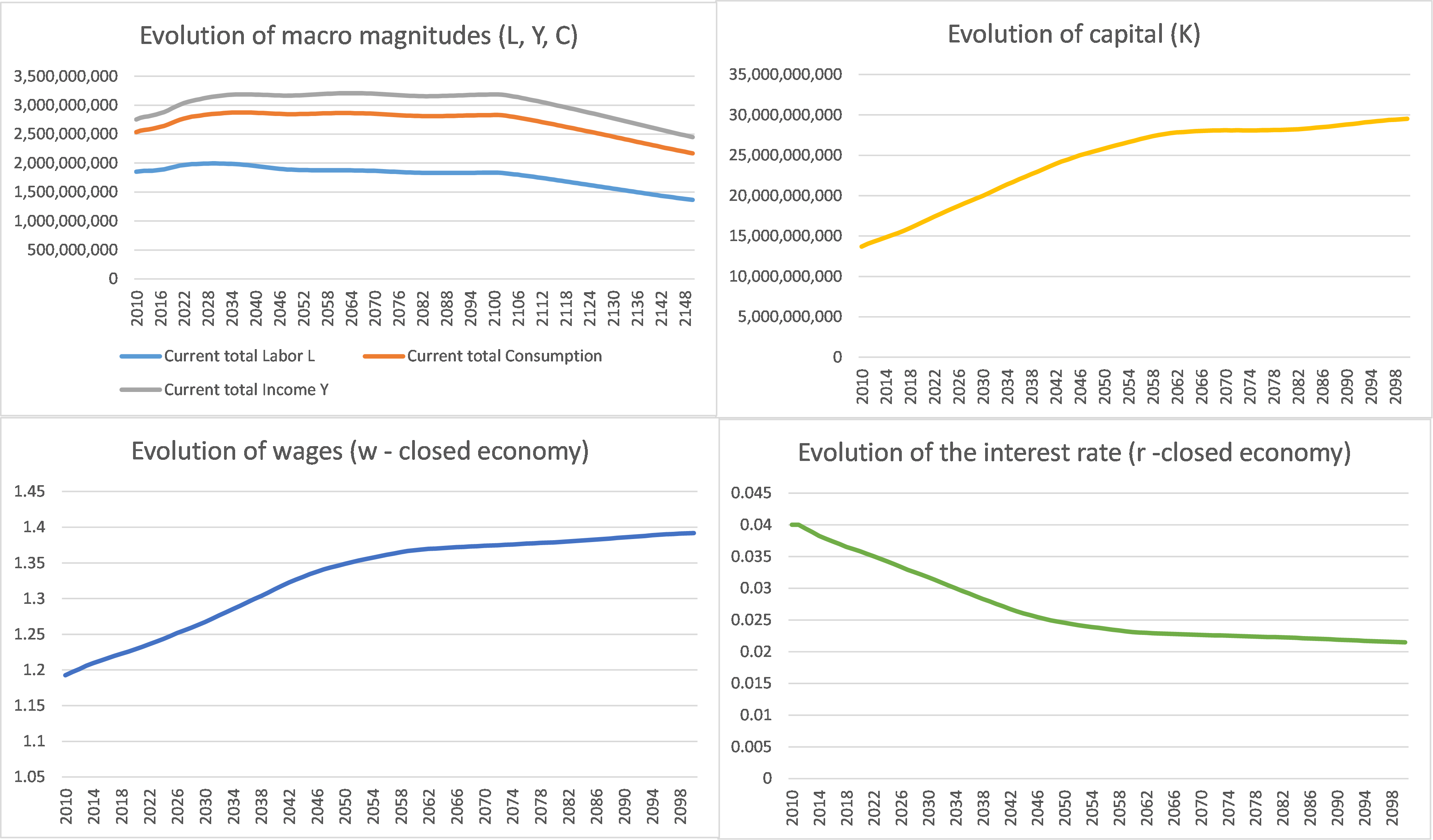
b) Austria (base scenario, closed economy)
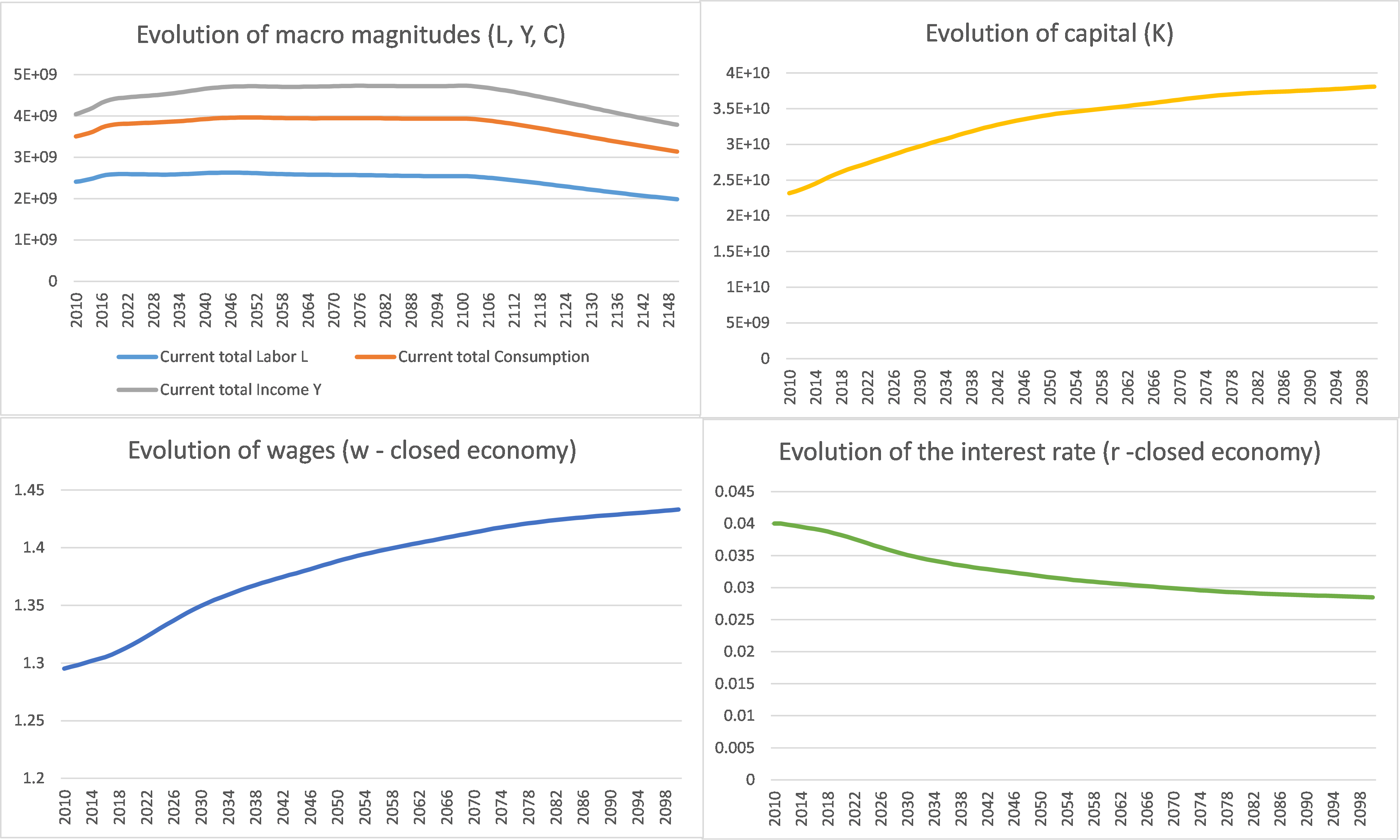
b) Finland (base scenario, closed economy)

b) UK (base scenario, closed economy)